Conservation of Energy:

    Like all things, Stirling Engines follow the conservation of energy principle (all the energy input is accounted for in the output in one form or another). The reality of the Stirling engine is that the input energy comes from the external heat source some is discarded at the cold sink, more is lost to friction, some is lost to sound, some to air drag on the flywheel, and the small bit remaining is the work output. To simplify the model we shall ignore all energy transfer besides that at the heat sinks and the the crankshaft.


Conservation of Energy for Heat Engines:

Conservation of Energy for a Heat Engine
Courtesy of askIITians.com

The Hot and Cold Reservoirs are the heat sinks mentioned in previous articles. The hot one supplies all of the energy QH, while the cold one removes energy QC (a necessary part of the cycle). The Heat Engine in question is the Stirling Engine, though many others exist that could be studied in the same manner. The arrow to the right in the diagram indicates work W (useful energy) is produced by the system.

Math:
So, now to the fun part! Again this only applies to the ideal Stirling Engine that functions without any non-conservative forces like friction. the following calculations will not include any numbers, but it will demonstrate how one could calculate the work, efficiency, ideal efficiency and ....


Equation:
 Description:

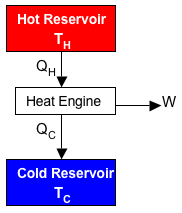
Conservation of Energy Principle

For a cycle, the initial and final state of the system are the same and thus have the same amount of energy.

Because there is no change in energy between the initial and final states of the cycle, all added energy must leave within the same cycle.

The only energy added to the system in heat from the Hot Reservoir.

Energy leaves the cycle at the cold reservoir and in the form of work. (Ideally only in those two forms, in reality it would include far more forms).

Rearranging the energy balance, these are all the ways in which energy is transfer to or from a Stirling Engine.

Solving the energy balance for work output, in terms of heat transfer in and out.

Another bit of information one might be interested in is the thermal efficiency (ηth) of the Stirling Engine.

Theoretically, the highest efficiency for a heat engine is the Carnot Efficiency, which can be calculated if one knows the absolute temperatures of the hot and cold reservoirs
Formulas re-produced with help from codecogs.com

From the above, one can, knowing a few values determine the work a Stirling engine can produce, how efficient it is and compare its efficiency to the maximum efficiency that a heat engine can have given the temperature of its' reservoirs.

The math above applies to all Stirling Engines, so if you'd like to learn about the variations, click here.