A rocket engine is very much similar to a balloon or a fire extinguisher in its basic principles and how it works. The fuel is pressurized inside of a contained device then released under high pressure out the opposite way they want to travel, similar to letting go a filled untied
balloon. This release of pressure creates a force as the fuel escapes the chamber through a relatively small opening, thus creating a force, and by Newtons third law there will be an equal force the opposite direction as well, accelerating the rocket. Thrust also uses the principle
of conservation of momentum, which states that momentum of a body in a closed system cannot be created nor destroyed, only transferred, and since the fuel has mass and velocity it has momentum which it transfers to the rocket as it begins thrust.
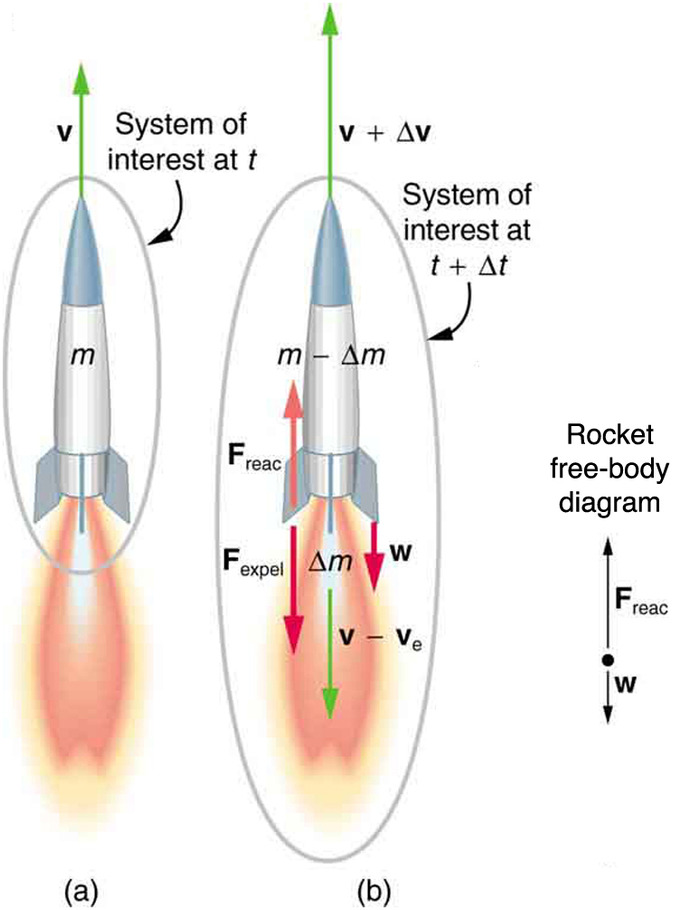
If we have a rocket coasting with an initial momentum(Pi) with a final momentum(pf) after it begins thrust, by conservation of momentum we can work out the thrust of the rocket. (Pi=Pf)
Eq (2). Pf=mf*vf
The mass initial and velocity initial would simply be the mass and velocity at the beginning before thrusting, but the mass final and velocity final would be a tad more complicated. At the moment after thrusting some mass has been used to generate some thrust, so we would have the final momentum equal to. Pf=-deltaMve + (M+deltaM)(v+deltav) where M is the final mass and delta M is the change in mass from initial to final and v and delta v are the same but for velocity.
Eq (3). Mv = -deltaaM(-ve+v+deltav)+(M+deltaM)(v+deltav)
Solving further will break this equation down to thrust in terms of burn rate and exhaust velocity.
Thrust = -(veR)
The Thrust will be as strong as its ability to expel fuel exhaust by the amount per unit time, which we can then put into numbers with some critical thinking, basic algebra, the conservation of momentum principle, or with some basic calculus.