 aquaviews.net
|
Buoyancy
is
important
to
a diver for a seemingly simple reason. If the diver is too buoyant, he or she will not sink, and instead of diving, will find him or herself snorkeling with a tank strapped on his or her back! What does physics tell us? ? |
Thank you, Archimedes!
Archimedes
principle states that an object immersed in a fluid is buoyed up
by a force that is equal to the weight of the liquid displaced by the
object.
tutorvista.com
tutorvista.com
 howstuffworks.com
|
For a moment, imagine how heavy
the iceberg on the left must be. We can think about how heavy a bag of
ice is when we carry it home from the store and then imagine how small
that bag of ice is compared to this iceberg. Yet, despite how heavy
this enormity must be, it floats! First, we consider the force pushing the iceberg down into the water. It is the same force that the diver at the top of the page experiences when he takes a giant stride entry off the side of a boat- the force of gravity. The force that you feel from gravity depends upon your mass. Intuitively, you already know this. Consider a rock and a pillow that are about the same size. Would you rather be hit by the rock or the pillow? I suspect that I am not alone when I say I would rather be hit by the pillow, but why? Well, being hit by the rock hurts more even though they are the same size. This is because the rock is more dense than the pillow, and therefore it has more mass. We can remember this with the following equation: m=d×v
That is, mass equals density times
volume.
We figure this into the force due to gravity, that is the force due to gravity equals the mass times the acceleration due to gravity. Fg=m×a
If the force of gravity is pushing
the iceberg down, why doesn't it sink?
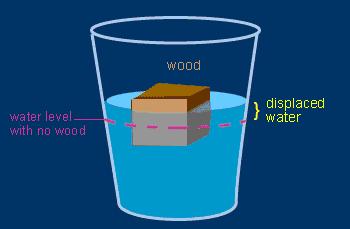
Recall Archimedes principle. The iceberg is displacing water and is therefore being buoyed up by the buoyant force. That is the volume of the liquid displaced, in this case water, times the weight of the water. FB=v×w When do a buoyancy check and find
yourself properly weighted, i.e. you don't completely sink when you
fill your lungs with air, the force of gravity pushing down on you and
the buoyant force pushing up on you are equal. When add weights to your
gear, you are increasing your mass greater than you are increasing the
volume of the water you displace and ta-da, you sink!
|