 |
|
|
Home
Page
A
little history about Kirchoff
A
little history about Ohm
Kirchoff's
current and voltage laws
Ohm's
law
Circuit
analysis example
Bibliography
The nitty-nitty-gritty, circuit analysis examples using Ohm's Law and Kirchoff's Current and Voltage Laws.
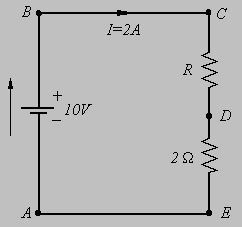
First lets look at a series circuit using Kirchoff's Voltage law and Ohm's law to find the value of the unknown resistor R. In the following circuit, it is known that a 2 ampere current flows in the circuit.
If we start at point A and create a clockwise loop around the circuit, the voltage at A is zero (or it can be considered ground). Then at point B the voltage must be 10 volts because the voltage supply creates a 10 V potential between point A and point B. At C the voltage is still 10 volts because points B and C can be considered the same points (voltage does not change along an ideal wire that has no resistance). However, going to point D, there is a voltage drop across resistor R , and another voltage drop going to point E across the 2 ohm resistor. Since points A and E are the same points, the voltages there are the same, zero, and our loop is complete.
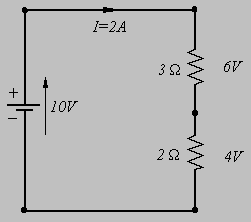
Now using Ohm's Law in the form V = I R, one can find that the voltage drop (I * R) across the 2 ohm resistor is:
(2 A) * (2 ohms) = 4 V.
Thus, by Kirchoff ' s Voltage Law, the voltage drop across the unknown resistor must be:
10 V - 4 V = 6 V.
Finally, using I = 2 A, and Ohm's law in the form R = V / I, the unknown resistance (R) is found to be R = 3 ohms.
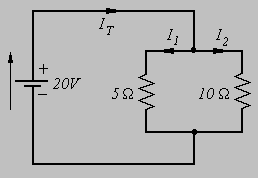
Next, lets solve a circuit using Kirchoff's Current law and Ohm's law to find the unknown current in the following parallel circuit. In the circuit, the source voltage is 20 V and the resistor values are 5 ohms and 10 ohms, respectively.
Because voltage does not change along an ideal wire that has no resistance, the full 20 V from the supply appears across each resistor. Next, using Ohm's Law in the form I = V / R we find:
I1 = 20 V / 5 ohms = 4 A,and:
I2 = 20 V / 10 ohms = 2 A,
Finally, by Kirchoff ' s Current Law I(total) is found to be:
I1 + I2 = ITIT= 4 A + 2 A = 6 A.
Thus, I1 is 4 amps, I2 is 2 amps, and IT is 6 amps.
