Brentley Powell - Black Hole Physics

Homepage  Fundamentals  Relativity  Thermodynamics  Resources


Relativity

        The concept of a black hole first publicly proposed by John Michell in 1783. He considered a classical gravitational field strong enough that the escape velocity was greater than the speed of light.
        
        "...[it] is therefore possible that the largest luminous bodies in the universe may, through this cause, be invisible."
- Simon Pierre LaPlace, 1796


Classical Black Holes

        A problem arises with the classical description of a black hole. As the mass collapses into a singularity, the light which it emits is still visible to the outside. Collapsing into an infinitely dense singularity which interacts with the external environment would require the release of an infinite amount of energy.


Classical, 'Newtonian' black hole with a naked singularity.
(from https://www.pitt.edu/~jdnorton/teaching/HPS_0410/chapters/black_holes/index.html)

        The first rigorous descriptions of a the region of spacetime beyond the event horizon of a black hole would not come until after Albert Einstein published the Theory of General Relativity. Karl Shwarzchild was the first to produce such a result when he solved Einstein's Field equations for the conditions of a black hole in 1916.

        The beauty of Einstein's General Relativity lies within its principles of conservation and equivalence. Einstein observed that two inertial frames are indistinguishable from one another without information coming from outside of one's frame. Similarly, He recognized that the force experienced by acceleration in free space is indistinguishable from the force felt remaining stationary in a gravitational field. Einstein had to formulate a description of space and time where the speed of light remained constant between both inertial and acceleratory frames. He determined that for conflicting frames to agree about the speed of light, space and time must be conserved as a unified space-time. The observed consequences of Einstein's conclusion are perhaps well showcased in the form of an anecdotal thought experiment.


        General Relativity Thought Experiment

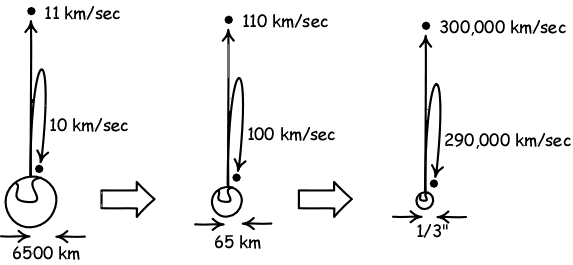
        Consider two aliens named Tik and Tok. Tik remains an arbitrary distance away from Tok, who decides to freefall towards the event horizon of a black hole. Tik and Tok remain within one another's line of sight, so they frequently exchange photons. As Tok accelerates toward the event horizon, Tik sees that Tok's photons have lost some of their energy to the gravitational field of the black hole. The photons have red-shifted to a greater wavelength. This is not what Tok observes. To Tok, Tik and the space he inhabits appear to have been compressed head-on so that his photon maintains its wavelength. He also observes that Tik's photons are blueshifted, gaining energy from the field. As Tok nears the event horizon, he sees time speed up for Tik while his own time remains steady. Tik now sees that Tok seems to be nearly frozen, fading to red, and stretched front-wise. Tok is now being bombarded by high-energy radiation as he observes Tik speed up, the space between them shrunk even further. It would have taken an infinite amount of time for Tik to observe Tok crossing the event horizon.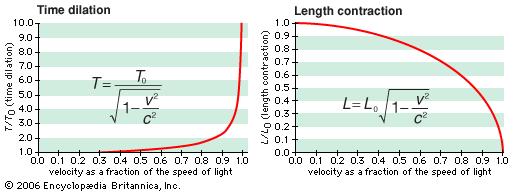


To Tok, Tik seems to dilate in time and contract in length as Tok is in an accelerated frame.
(from from https://www.britannica.com/science/time-dilation/media/596118/86705)

Other Properties of Black Holes
        According to the No-Hair Theorem, black holes do not have any variable characteristics other than position, momentum, mass, angular momentum, and charge. All other properties of black holes relate to constant factors of these three physical values. The nature of statistical distributions makes the probability that a black hold carries a significant charge very low. However, Angular momenta of black holes can be quite extreme. Some black holes have been found to rotate at a speed greater than 84% of the speed of light.


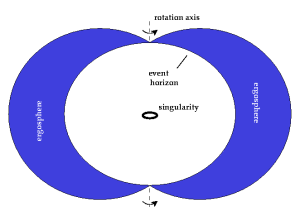Rotating black hole with ring-singularity.
(from https://galileospendulum.org/2011/09/02/some-further-notes-on-black-holes/)

        Angular momentum causes rotating black holes to be slightly squashed along their axis of rotation. The effect is that rotating black holes have multiple horizons, and a ring-like singularity rather than a point. The inner horizon acts as the event horizon. Between the inner and outer horizon, objects are forced to co-rotate with the black hole. Within the outermost region, the ergosphere, angular momentum is also transferred through the relativistic frames of objects outside the horizon. Such objects are accelerated radially as they are dragged through space. Rotational energy can be extracted from the rotational energy of such a black hole.


See Resources for full list of sources.