[ http://www.earthshots.com/images/Thumbnails/WholeEarthThumbnail.JPG
]
[ http://www.earthshots.com/images/Thumbnails/WholeEarthThumbnail.JPG
]
The Foucault Pendulum is not only a novel curiosity, but also a device that enables us as humans to provide some veracity to our model of the universe. It has been used in historical as well as contemporary contexts to validate the Newtonian concept of the world. It is an obvious visual result that we are standing upon a rotating earth, and following this, that the heliocentric model is correct. Not that this is in much dispute these days, but you can use it in explanation to unbelievers, and it won't sound like black magic.
In considering the physical workings of the Foucault Pendulum, it is helpful to examine the changes in velocity and acceleration in a rotating coordinate system, a pertinent example being our planet, in which reside all of our laboratories. Although Earth is a highly relevant example, we shall first examine the general case. The general case, in which the mathematics becomes apparent, is one where an object is tumbling about all three Cartesian axes. To picture this rotation, imagine that you have just tossed a ball of some kind; just as you were about to release it from your fingers you gave it a slight twist of the wrist, and let it roll off your fingers with a little bit of drag so that there is a rotation about each axis. Now, imagine that by some miracle, the rate of rotation is the same about each axis. In that case, the axes and their deviations should resemble the illustration below:
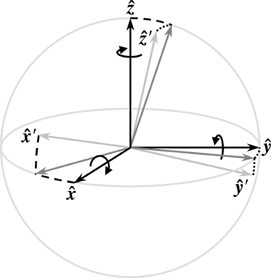
Derivation [1]
All 
's
are equal in magnitude, if not direction.
You will notice that in the deviation from
to
there is a change in the 
direction and in the 
direction. We are going to call this distance 
.
Each of these is a small measure of arc length, and by the relation
we get 
.
Since 
,
we can say that 
.
Since we are using 
for an infinitesimal change in arc, we will note that its vector
magnitude is one, making the relation 
.
This relation only describes the change in one component of the arc
length (
direction). The other arc length component, in the
direction, has the relation 
.
This produces the final
relation: 
.
This designates the deviation in time of a vector originally along
the -axis
as a result of rotating around the 
and 
axes.
Performing this same process for each axis, we
find that 
,
and 
.
(R1)
Now let's consider a vector 
(R2)
Noting that both the magnitudes and the hats are
functions of time, we will apply the product rule of calculus when we
take the derivative:
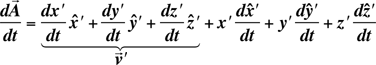(R3)
Recalling what dx'hat/dt etc. are defined as, this
results in:
(R4)
With some examination, we now note that this is
the cross product of
and 
.
Thus we have: 
(R5)
Holding this result to be true for any vector in a rotating coordinate system, we'll take a further time derivative to find the acceleration.
Let's look at inertial vector ,
where:
For the sake of avoiding errors, we will be very
explicit in the mathematical details.
(R6)
(Again from the product rule of calculus, keeping the rotational part separate to clarify the next step.)
(R8)
(R9)
(Recalling that you are taking the derivative of
(R5),
the original equation, you perform the same operation on
(R7)
as was done on the vector
that resulted in (R5).
You also insert (R5)
into the first cross product.)
(R10)
(Distributing the cross product.)
The result is 
(R11)
(Adding like terms.)
References
[1] Mechanics: Third Edition, by Kieth
R. Symon, page 277
(R7)
