Maximizing the Squat
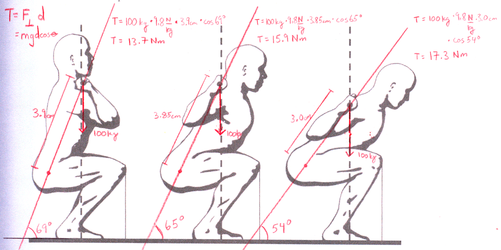
This drawing illustrates how Newton's Second Law and the concept of Torque can be used to maximize muscular efficiency in the squat.
We can also use this information to determine the moment of inertia.
We can also use this information to determine the moment of inertia.
In powerlifting, the goal is to move the most amount of weight possible. Because the muscles of the hip, particularly the gluteus maximus, are the the largest muscles involved in the squat, a bar position is chosen that places the greatest amount of force on this area.
The bar placement of the front squat decreases the moment arm of the hip the most of all three bar placements.
The low bar back squat is most commonly used in powerlifting as it has the greatest hip moment arm.
|
The back squat decreases the moment arm towards the knee and places greater emphasis on the hips.
|
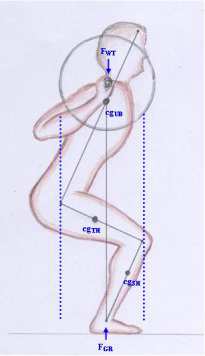
Power Example
This free body diagram shows the forces involved in the squat movement. For the purposes of determining power, we will use the following values:
Solved Below
This free body diagram shows the forces involved in the squat movement. For the purposes of determining power, we will use the following values:
- m = Mass of load = 150kg
- d = Distance travelled = 0.96 meters (note: this value is for only one direction of movement and will be doubled to determine the total distance that the load must travel)
- g = Gravity = 9.8 m/s^2
- t = Time to move load = 2.2 seconds
- a(d) = acceleration of the load in the downward direction = 1.2 m/s^2
- a(u) = acceleration of the load in the upward direction = 0.8 m/s^2`
Solved Below
Lowering
W = m(g-a)d = 150kg(9.8m/s^2 - 1.2m/s^2)(0.96m) = 1,238.4 J
P1 = (1,238.4 J)/(2.2 sec) = 562.9 Watts
Raising
W = m(g+a)d = 150kg(9.8m/s^2 + 0.8m/s^2)(0.96m) = 1,526.4 J
P2 = (1,526.4 J)/(2.2 sec) = 693.8 Watts
Total Power
P1 + P2 = 562.9 W + 693.8 W = 1,256.7 Watts are exerted by the lifter in lowering and raising the load.
This is a very simplistic determination and fails to take into account the variable levels of acceleration that occur at different points throughout the lift. It also fails to take into account the work done to move the lifters' own body weight. However, it gives a basic idea of the amount of power exerted by a lifter performing a squat.
W = m(g-a)d = 150kg(9.8m/s^2 - 1.2m/s^2)(0.96m) = 1,238.4 J
P1 = (1,238.4 J)/(2.2 sec) = 562.9 Watts
Raising
W = m(g+a)d = 150kg(9.8m/s^2 + 0.8m/s^2)(0.96m) = 1,526.4 J
P2 = (1,526.4 J)/(2.2 sec) = 693.8 Watts
Total Power
P1 + P2 = 562.9 W + 693.8 W = 1,256.7 Watts are exerted by the lifter in lowering and raising the load.
This is a very simplistic determination and fails to take into account the variable levels of acceleration that occur at different points throughout the lift. It also fails to take into account the work done to move the lifters' own body weight. However, it gives a basic idea of the amount of power exerted by a lifter performing a squat.