It probably comes as no surprise that acceleration plays a large role in cross country skiing. Rounding a corner, or rather trying to round a corner, while skiing down hill can often lead to disaster. On the other hand, decreasing acceleration (or deceleration) can also cause problems. Imagine a skier accelerating only to be tripped by a hidden tree root.  The skier's acceleration would greatly decrease as the ski stopped on the root, and there is a possibility for injury.

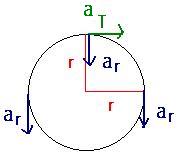
One type of acceleration experienced by skiers is linear acceleration. This acceleration is simply the final velocity minus the initial velocity divided by the difference in time. Constant acceleration = (V f -Vi)/(Tf-Ti) . This means that if a skier starts from rest and 2 seconds later the skier is traveling 4 m/s, the skier is accelerating at 2 m/s ² .

Circular acceleration is another aspect of skiing. There are two components of this acceleration, radial and tangential.  Below is a circle with some of the radial and tangential acceleration vectors drawn in.

Radial acceleration can be found by dividing the velocity squared by the radius. Radial acceleration = ^v2 /_r .  Radial acceleration occurs because of a change in direction of the velocity.  From the formula above it is easy to see why it is harder for a skier to make a turn with a small radius than a turn with a large radius.  Due to the fact that the radius is in the denominator, the smaller the radius the greater the acceleration and the larger the radius the smaller the acceleration is.

Tangential acceleration = d|v| / dt.  Tangential acceleration is what causes a skier to change speed while rounding a corner.  The tangential acceleration plus the radial acceleration are equal to the direction of the acceleration vector.