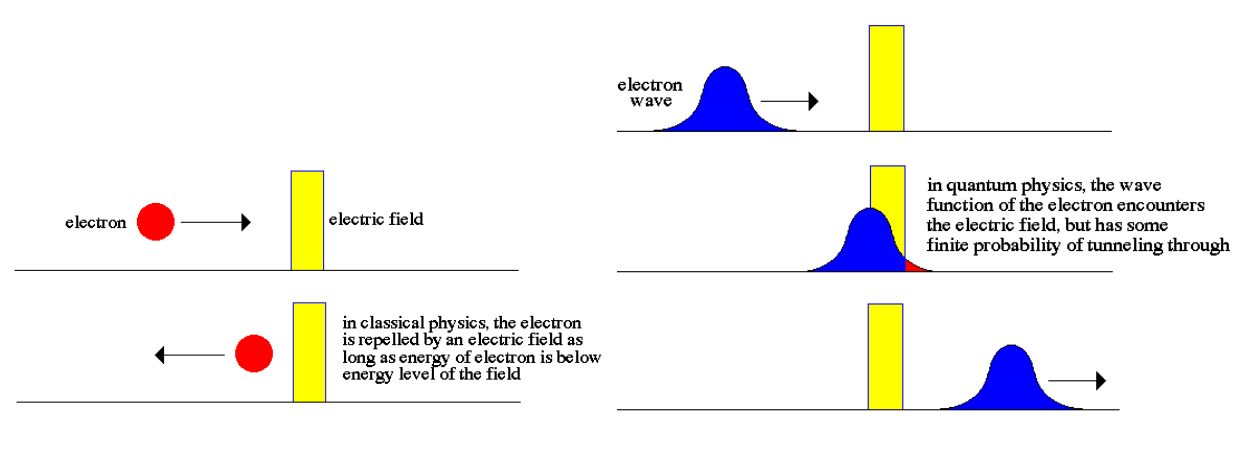
Electron tunneling is the non-intuitive process of an electron passing through a barrier that is usually deemed to be impassable. To understand this (well, kind of - see above), you need to remember that matter exists as both a particle and a wave. Remember? Awesome.
    Now picture yourself at the base of a steep hill. In front of you is a ball. For reasons unknown, you want to get the ball on the other side of the hill, but lack the energy to push it over the top. From a classical (Newtonian) physics point of view, that's it. Party's over. If you don't have the strength to push the ball over the hill, then the ball will stay on this side of the hill.
    However quantum physics allows for a more subtle approach: matter exists as both a particle and a wave, right? You remembered! Now in the previous situation, we talked about the ball as made up of many small particles. Carbon atoms forming long polymer chains forming a rubber ball. But if matter is both a particle and a wave, then this ball must also exist as a wave function. At this point, you should probably scroll up and reread the first quote: "Nobody knows how it can be like that," so don't worry. If this is true, and it is, then this wave-function ball can interact with the barrier in a totally different way. Instead of requiring a certain quota of energy to push it up over the top of the hill, the ball can travel through the hill. It can tunnel.
  
    At this point, it might be helpful to have a visual:

           Particles of matter exist as probabilities described by a wave function. The probability of finding a certain particle in space is higher at the peaks and troughs of the wave. The lower axis illustrates this by darkness of coloration: areas where the ball is dark are high probabilities, whereas areas where the ball is light are areas where the particle has a low probability of being found.
           The Heisenberg Uncertainty Principle (don't worry), states that the more precisely you try to pin down a particle's location or momentum, the more elusive its location or momentum become. You can know one thing, but you can't know more than one thing at one time. This can be used for a bit of trickery: locations of the particle exist as probabilities, therefore the particle has a non-zero probability of being anywhere. Some locations may be exceedingly improbable (approaching infinitely improbable), but there's still a chance it will be there.
            So, back to our hillside: though the ball may lack the energy to surmount the hill, there is another way. Though very small, there exists a probability that when the ball encounters the hill (remember the ball's a particle and a wave) it will not roll up the hill, but will instead 'exist' on the other side by means of probabilities. It will pass through the hillside without appearing to have physically done so. For lack of a better term, this process is called 'tunneling.'
            Don't worry if this doesn't make sense (scroll up and re-read the quotes), just know that particles such as electrons can pass through barriers by some tricky methods and that the mechanism for doing so is a very strange one.
                                                               (cc) Maschen
    Fig 1. Matter existing as both a wave (upper axis),
    and a probability (lower axis). Opaqueness of the
    particle on the lower axis illustrates the probability
    of existence (dark = more probable).