Buoyancy

Animals are denser than either fresh or sea water, and therefore tend to sink, unless they have adaptations that give buoyancy."                                                                                                                                             (R. Alexander, as qtd. in Reynolds)

As you know, if you throw a stone in the water, it will sink. In fact, anything that's denser than water will sink. You've probably noticed that you yourself also sink unless you expend energy and do work swimming to keep yourself afloat. So how come we see whales & manatees leisurely "floating" around, at whatever depth they want, not expending energy swimming but still not sinking? After all, they're heavier than us aren't they? The key, as we will see in a moment, is density.

First, lets explore the concept of buoyancy. What determines whether something floats or sinks?



As you could see in the TVOntario video above, whether something sinks or floats in water is determined by its density (mass/volume) relative to the density of water.
The density of water is 1 kg/L (this means that 1 liter of water weighs 1 kg), anything denser than that (that is, anything that has more mass per unit volume, so 1 liter of it ways more than 1 kg) will sink; anything less dense than that will float, and anything that is exactly 1 kg/L will be submerged motionless, without sinking or floating.      (Giancoli 263)

Why is this? Because of Archimedes' Principle
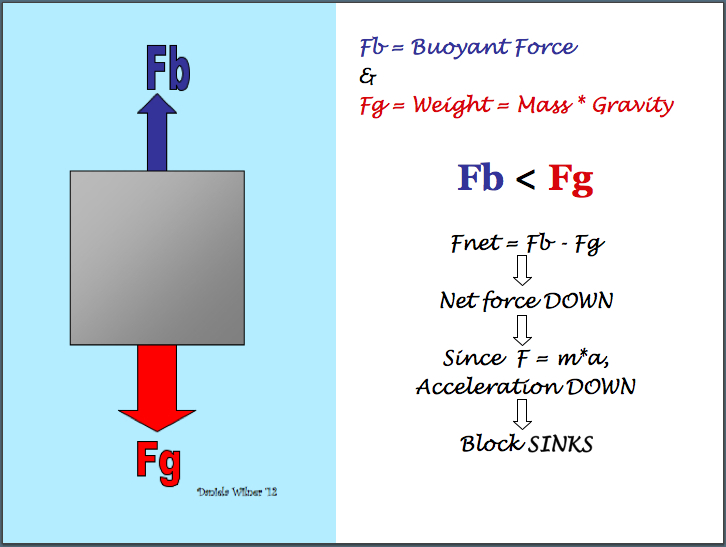
Archimedes figured out that "the buoyant force on an object immersed in a fluid is equal to the weight of the fluid displaced by that object" (Giancoli 263). Going back to the video, remember that since all the cubes were the same size (had the same volume), they displaced the same amount of water, so "the weight of the fluid displaced" by each one was the same; therefore the buoyant force acting on each one was the same. But each cube had a different weight, so even though the force pushing up on each cube was the same, the force pushing down on each one (mass*gravity) was different, resulting in the 3 different outcomes. Let's draw the free body diagrams.

• For the metal cube, the force of it's weight is greater than the buoyant force, so there will be a net force down, and the cube will accelerate down (sink).

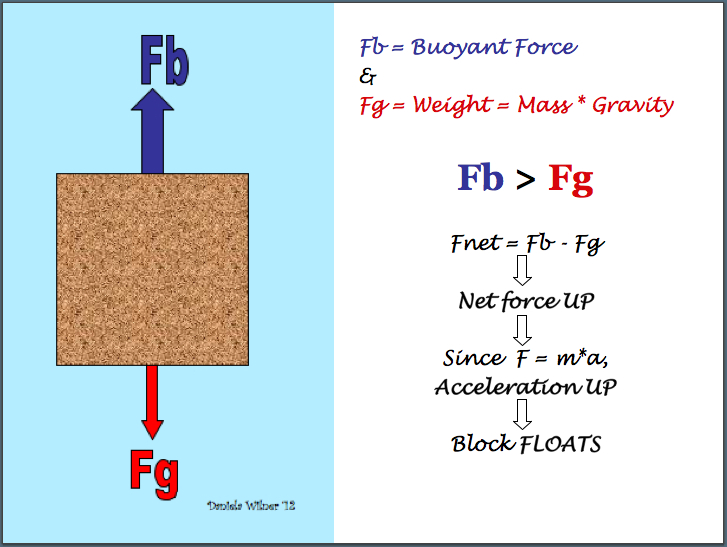
• For the cork cube, the force of it's weight is smaller than the buoyant force, so it will accelerate up (float).

• And for the plastic cube, which had the same density as water, the force of it's weight is equal to the buoyant force, so there is no net force acting on it, and therefore the object will not accelerate in either direction (because remember that F=ma -force=mass*acceleration-).

This last scenario is called neutral buoyancy, and it's what marine mammals have, so it doesn't take energy for them to stay still in the water, and it's not particularly hard for them to go up or down when they want to either. Imagine an animal that is as dense as a rock trying to swim up for air, or one that has low density, like a balloon, trying to dive down to find food; it would take a lot more energy to counteract that net buoyancy-weight force (up or down) than it would if that net buoyancy-weight force was 0. So by having neutral buoyancy, marine mammals save energy.
And how do they achieve neutral buoyancy? They have more fat in their bodies (fat is less dense than water), it also helps that saltwater is a little more dense than pure water, so the buoyant force is a little stronger.                                         (Horstmann-Dehn)