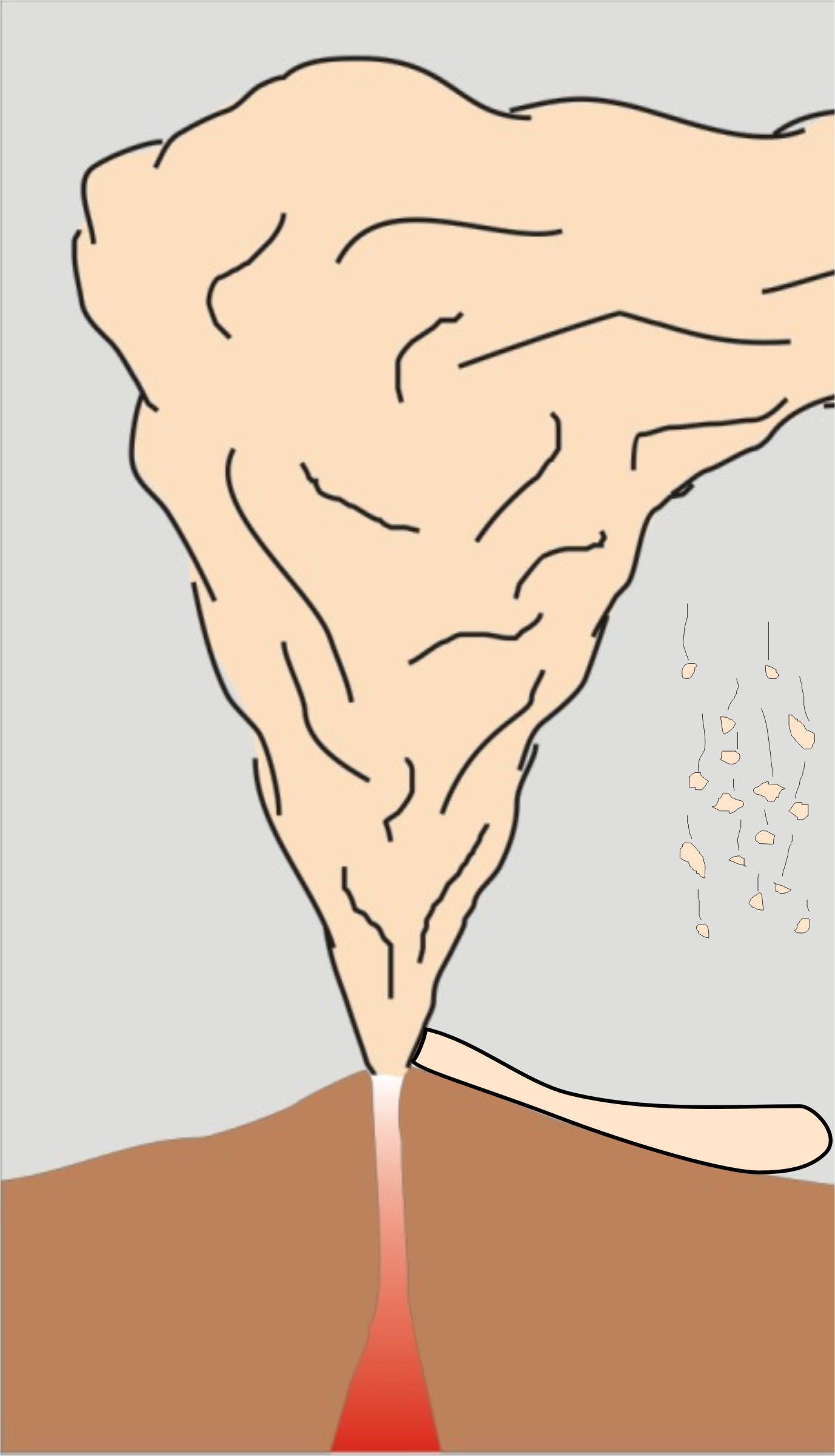
Buoyant Regime

The plume entrains air due to the high degree of shear between the Jet and the surrounding air. This shear is set up along the density contrast at the margin of the jet, and occurs as a Kelvin-Helmholtz instability.

How fast is air entrained?

The entrainment of air occurs turbulently along the margin of the plume, and propagates inward. If the plume does not become buoyant before the jet reaches a velocity of 0, then the plume will become non-buoyant, and form pyroclastic density currents.

Because entrainment is a turbulent process, the details are VERY hard to model. however, a statistical approach effectively predicts the rate at shich air is entrained. This technique shows that air is entrained, E, at a rate proportional to the Velocity, V, and an entrainment constant, K.

E=kV

Sparks, 1986

What makes the system buoyant?

There are two processes that need to be examined when considering buoyancy in the plume: adiabatic versus thermal rise and expansion. Really, the expansion is the crucial part of both mechanisms, for it is expansion that lowers density, allowing a parcel to rise.

Adiabatic Rise and Expansion

Let us consider a parcel of air entrained at the base of the plume. The jet will carry this parcel upward. This rise will lower the pressure on the parcel and allow it to expand. This expansion is described by

Where Vis the volume of the parcel at height h, Vo is the initial volume of the parcel, po is the initial pressure, p is the pressure at h, and phi is a ratio of heat capacities at at h and the initial height.

From Sparks, 1986

Thermal Rise and Expansion

But we also need to consider how the heating of entrained air by the plume will affect buoyancy.

Upon reaching height Hb, the height of neutral buoyancy, the height of neutral buoyancy, each parcel of air will have a temperautre contrast, Which can be descibed by the first of the equations below.

Where T is the temperature of the parcel at Hb, and To is the initial temperautre. Additionally, the parcels will expand from heating by the column. The second equation above whose the overall expansion induced by this heating. Vc is the thermally expanded volume, V is the adiabatically increased volume, Theta c is the center-line plume temperature, and Theta Hb is the adiabtic temperature at Hb.

Sparks, 1986

What happens to an expanding column?

Though expansion is significant, it does not result in an additional upward expansion of the column. Instead the parcels largely expand outward.

From Sparks, 1986