
A tire swing work a bit like a regular swing, except more complicated. Now we are going to introduce new forces that have to do with the spinning path that a tire swing takes. Once a force is applied, centripetal force (the force required to keep a body moving in a circular path) and centripetal acceleration (the acceleration of a body moving in a circular path) cause the tire to spin in a circular motion.
Uniform Circular
Motion
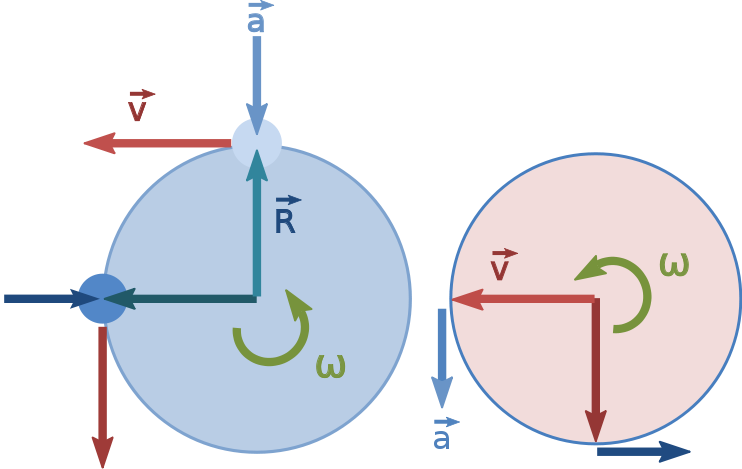
Now, if the tire swing traveled in a uniform circular path, you would move in a circle and the velocity would be tangent to the orbit as the blue circle shows. The red circle shows that acceleration is radially inward.
NonUniform Circular Motion
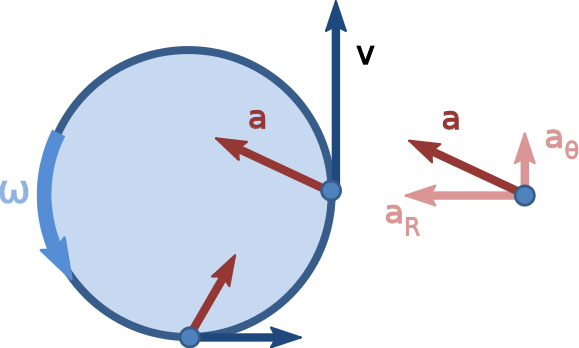
A tire swing moves more in a nonuniform circular motion, where the velocity is tangential to the orbit and the acceleration is not radially inward and the tangential component increases the rate of rotation.
Return to Main Menu