Geothermal Energy

Heat Engines:

Geothermal Energy in itself acts similar to that of a perpetual heat engine much like a sterling engine. With any geothermal heat pump you have a heat source and a cold sink. The cooler water or refrigerant flows through the system under the ground or into a body of water were it reaches the heat source (the earth, hot spring, or lake). The heat from the surrounding ground or water raises the temperature of the fluid flowing through the pipes which then returns to the home, building, or power plant. Depending on the system the fluid either gets vaporized to turn a turbine or goes directly into heating or cooling a household via a condenser.

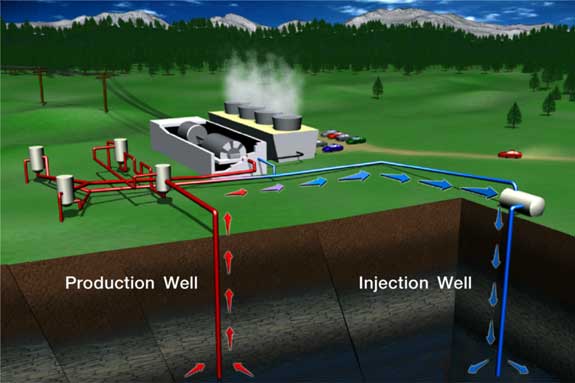
Heat cycle of a geothermal power plant

Efficiency:

When it comes to the efficiency of heat pumps there is really nothing that can beat them. If you take into consideration the total net thermal efficiency of a geothermal system which includes the efficiency of electricity generation and transmission then the average efficiency turns out to be around 40%. When you look at just thermal transmission though it is not uncommon to see efficiencies well above 100%. This is due to the fact that heat pumps move up to five times more heat energy than electric energy that the system consumes leaving the system with a surplus of energy.

Below is a sample calculation of the theoretical efficiency of a heat pump based on the mean average surface temperature of 15°C and a geothermal well drilled to 1000m with a temp gradient of 30°C/km.

e=efficiency
Q_H=Heat of hot source
Q_C=Heat of cold source

e= 1 - ((Q_H- Q_C)/ Q_H)

e= 1 - ((318K-288K)/ 318K)

e= 1 - 0.09433

e= .906 » 90.6% efficiency

This level efficiency assumes that no heat is lost to the environment which is bound to happen.

The coefficient of performance (COP) translates the energy performance of a heat pump. The COP of a heat engine can be expressed as the ratio between the amount of heat energy it produces and the energy consumed in order to fulfill the energy transfer.

The theoretical COP can be expressed by the formula:

COP_Carnot= T_C / (T_H - T_C)

where

T_H = Temp. of hot source in Kelvin
T_C = Temp. of cold source in Kelvin

Using the same conditions as with the efficiency calculation:

COP = 288K / (318K - 288K)

       = 288K / 30K

       = 9.6

This calculation is idealized and assumes there is no heat lost to the environment. The COP also depends on the size of the operation and the type of heat emitter that is used. The COP for most geothermal systems ranges in between 2.4-5.