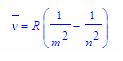
Prior to 1913 all experiments that were examining the electronic structures of atoms involved measuring the frequencies of electromagnetic radiation that are absorbed by atoms. Each of these particular patterns of emission are unique for each particular element. Rydberg took the emission lines and found that each individual emission line has a particular wave number associated with it. The wave numbers have units of inverse centimeters, (cm-1).
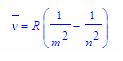
m = 1 is known as the Lyman series and it converges to 109,678 cm-1. m = 2 is the Balmer series. Each value for m, converges to a different value. n = m +1, m+2, m+3… The Rydberg constant is found to be 109,678 cm-1.
In 1913 Neils Bohr, a Danish physicist reasoned that the Rydberg equation can be explained in terms that only discrete frequencies could be absorbed or emitted by an atom. Each electron would have discrete energies.
Bohr proposed that the electrons orbited the nucleus indefinitely in a fixed radius. According to the physics at the time this was not possible, it was thought that the electrons would start to spiral towards the nucleus, in doing so there would be infinitely many frequencies for each position of the electron as it follows a path that will eventually crash itself into the nucleus.
For each one of Bohr’s discrete orbits, the angular momentum must be quantized.

n is an integer representing the quantum number, m is for the mass, v is velocity, r is the radius, and h is Planck’s constant. The radius could be found by,

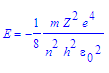
Bohr later found that empirically energy was also equal to,

French physicist Louis Victor de Broglie in 1924 said that all matter had wavelike properties.

Two
years later two Americans
stated that de Broglie was correct.
The Viennese physicist Erwin Schrödinger described the electron in way that emphasized the wavelike behavior. In the most general form, the Schrödinger equation is,
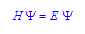
H represents the Hamiltonian operator. The Hamiltonian operator describes a series of mathematical equations that are for the wave function, Ψ. The wave function describes the wavelike properties of the electron. E is the energy that the electron could potentially have if it behaved in the manner described in the wave function.
Some hydrogen-like wave functions Ψ = R(r)Θ(θ)Φ(φ)
1s
orbital 
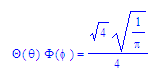
2py
orbital 


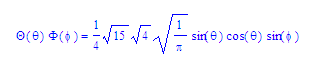
Nc, a, and b are all dependant on one or more of the quantum numbers m, l, and ml. a0 is the Bohr radius. Equations are set up to be plotted in a spherical coordinate system.