acting on it, while being inversely proportional to its mass.
More often though we see this law written as;
Fnet = ma
Where "F" equals the net force on the object in Newtons, "m" equals the mass in kilograms and
"a" equals the acceleration of the object in meters/second^2. Since force is a vector this can be
rewritten in three separate equations to take into account direction;
Fx = ma, Fy = ma, Fz = ma
Fnet = Fx + FY+ Fz
We must also remember that acceleration as well is a vector and therefor has three components just
like the force. When learning how to use Newton's 2nd Law, however, one usually only uses examples
where there is only two directional forces acting on an object. These are usually the x-direction (side
to side) and the y-direction (up and down). For now we are only going to take into account these two
components.
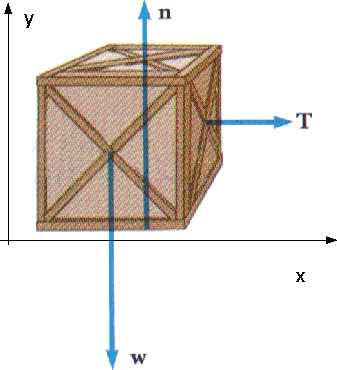
http://oldsci.eiu.edu/physics/DDavis/1350/05Laws/intro.html
In the diagram above we can see the two axis, x and y. From this picture all three forces are exactly
parallel to the axis. This means either the force is all in the x-direction or all in the y-direction. Because
we do not have to figure out how much of each force is acting in the x-direction and how much in
the y, this is one of the most basic problems. From this we will get the two following equations;
Fx = T, and FY = n + (-w)
From this we can than determine the acceleration of the object in the x-direction and also then in the
y-direction.
Fx = T = max
ax = T/m also described as the horizontal acceleration
FY = n + (-w)= may
ay = (n - w)/m
Using common sense though we can also see that the accelerating in the y-direction is going to be
equal to zero since we know the box will not just jump up all the floor or fall into the floor. Therefore
since there is no force other than the normal force (we will talk more about this in discussion about
) and the force due to gravity we know that these will equal leaving us with;
ay = 0
This give us;
anet = T/m in the positive x-direction.
This law is very useful in the fact we can determine the acceleration of an object if we know the forces
acting on it as well as it's mass. Turing our thinking the other way, we can also find the forces acting
upon an object if we know it's acceleration and mass. The main thing we must remember is that forces
as well as acceleration are both vectors and therefore have directions. Once we have found the horizontal
and vertical components, however, we then use the same equations as in the above example.
Background image from: http://www-gap.dcs.st-and.ac.uk/~history/Mathematicians/Newton.html