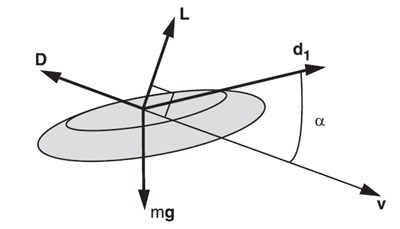
The reason for the prolonged flight of a Frisbee comes down to Angular Momentum.
When a Frisbee is thrown, it is thrown with a spin. This spin gives the Frisbee angular
momentum. Angular momentum(L) depends on moment of inertia(I) and angular
velocity(w).
Angular Momentum
L = I*w
http://www.sciencebuddies.org/Files/2265/5/Aero_img016.gif
To increase angular momentum, either increase the moment of inertia, or increase
the angular velocity. In other words, you can either distribute the mass of the Frisbee
further toward the rim or throw the Frisbee with faster spin.
The moment of inertia
is given by multiple expressions for different shaped objects, such as disks, spheres,
cylinders, hoops, slabs, and so on. If the Frisbee had even distribution of mass its
moment of inertia would be (1/2)*mass*radius^2. If the Frisbee more closely
resembled a hoop with its mass distributed right at the edge, its moment of inertia
would be mass*radius^2. Obviously there is an advantage to having the mass distributed
more closely to the rim if a Frisbee with higher angular momentum is desired. This is
the logic behind the Aerobie Ring (pictured below). These types of discs travel very far and very
straight because of their high degree of angular momentum. The angular momentum maintains their stability
lending to more control and farther throws.
Angular velocity is a variable that is controlled by the thrower.
If thrown with high spin (angular velocity) the Frisbee will be more stable and a flatter, straighter flight path with result.
Higher angular velocity is obtained through the wrist during the throw, not the shoulder. If a good
whip or flick is given at the end of the throw, the disc will spin more quickly and be more stable.

http://aerobie.com/ProductInfo/WebImages/Alones/Sprint-Yel-ForWeb350.jpg
Home History Airfoil Flight Bibliography