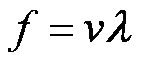
Cool Math Observations

Frequency, wavelength, and amplitude

• Frequency: The number of cycles per second a complete wavelength passes through the length of an instrument. The frequency also determines the pitch of a note.
• Wavelength: The distance between wave crests (sinusoidal representation)
• Amplitude: The height of a wave from centerline to crest. The amplitude determines the volume of a note.
• Fundamental tone: The tone carrying the longest wave and the lowest frequency in a harmonic row.
• Overtone: Subsequent tones that follow after a fundamental tone in agreement with the harmonic row.

Frequency = velocity*wavelength relationship

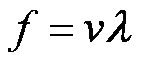
In brass instruments, if frequency is the produced pitch of a note, then velocity is the pressure a player applies through their air and wave length is the length of the longest wave that will fit into a chamber.

The Harmonic Row

Observe, in the photos, the demonstration of this relationship.

The observation begins with the player in 6 th position (almost longest bore of horn). As the player increases the air velocity, he produces higher and higher notes—overtones. These notes are members of the resulting row of harmonics specific to this slide position. The wavelength remains constant, but the velocity is increased. Hence, the frequency, and thereby pitch, increase by simple harmonic ratios with the fundamental.

The player then reduces the length of tubing, and thereby the wavelength as well, to 5 th position. The player applies approximately the same pressure to create the second row of harmonics. This row of harmonics is approximately one half-step above the first row of harmonics from 6 th position.

Each successive reduction in tube length decreases the wavelength drives the frequency higher and into a higher row of harmonics.

Twelfth root of two

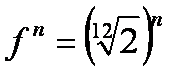
The twelfth root of two is the constant ratio that, when raised to incrementally increasing integer powers (n) and multiplied by a fundamental frequency (i.e. 220, A below Middle C), the product frequency increases in pitch chromatically along the 13 tone scale.

The distance between each of the thirteen harmonics per scale is exactly Hz: