Kepler’s Second Law

The idea that Kepler sought most in his dealings with astronomy was a theorem that would have the capacity to predict where a planetary body would be at a particular time. This, of course, would require the knowledge of the velocity of the body at any point in its orbit. The key to solving this problem is finding the relationship between the different velocities of a planet as it travels through its orbit.

Kepler’s solution to this problem involved making several assumptions.

1) All planets are driven by a force emanating from their axis of rotation, in other words, the sun. Although we now know this force to be gravity, Kepler viewed it as a ray coming from the sun and acting of a planet as a paddle acts on a boat.

2) This force would decrease at a rate of the inverse of the radius from the orbit. Although we now know this to be the inverse of the square of the radius, Kepler viewed the force acting on a two dimensional plane, thinking that a force would not be present where there was nothing to act on.

3) The speed of a planet is proportional to the force pushing it and hence, according to his first assumption, inversely proportional to the distance.

4) The sum of the distances from the sun to the planet is equal to the area swept out by a line from the sun to the planet during a single period.

5) The orbits of the planets are perfectly circular. Although this assumption is not accurate, as Kepler later found out which the discovery of his first law, it is a close enough approximation to the actual orbits.

With these assumptions about planetary movement, Kepler was able to derive his second law, stated formally:
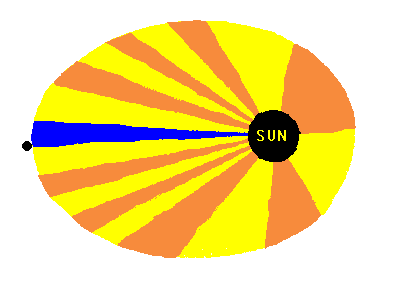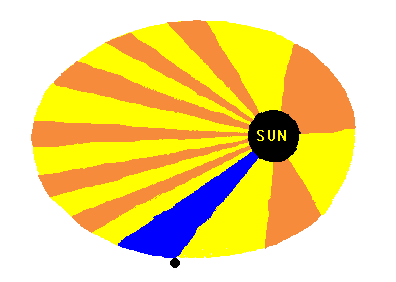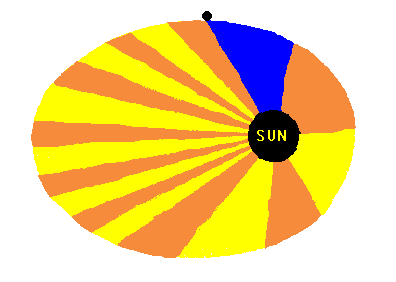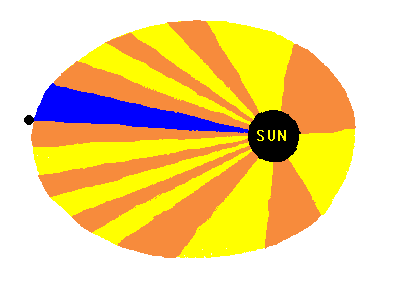
During a given time interval a line drawn from the planet to the sun sweeps out an equal area anywhere along its path.

http://home.cvc.org/science/kepler.htm

The key conclusion that can be determined by this law is that the angular speed of a planet is constantly changing as it moves around a sun. For instance, when a planet further away from a sun, the speed at which it travels is slower, and, conversely, as the planet moves closer to the sun, the speed increases.

For an interactive simulation for how the eccentricity of an orbit affects the speed of a planet, go here: http://www.sunsite.ubc.ca/LivingMathematics/V001N01/UBCExamples/Kepler/kepler.html