The Physics Behind Precession The gravitational interactions with the Moon and the Sun produce a torque on Earth that results in centripetal acceleration. Equation: The Sum of the Torques = Inertia x Angular Acceleration . Isaac Newton's Second Law of Motion states that a force exerted on a body will result in an equal change of momentum over a certain time. This is the conservation of momentum principle. Since the greatest pull is on Earth's bulge, the rotational axis points away from the combined vectors of the torques. These forces vary with distance, yet Earth's axis seems relatively fixed in space; it only varies between 21.5 and 24.5 degrees in obliquity with a frequency of 41 000 years. This then means that as Earth keeps its rotational plane steady, the sum of the net forces must be zero. Since Earth has a large stable rotational inertia due to its relatively high angular velocity, the inertia will be constant, and a change in angular momentum as Earth precesses can therefore only be produced by a small change in velocity (Hecht). Equation: Angular Momentum = Inertia x Velocity (L=I x w). Precession occurs with a change in angular momentum. 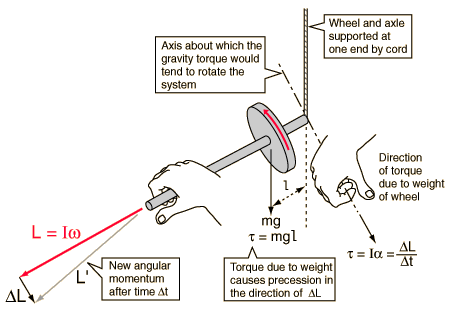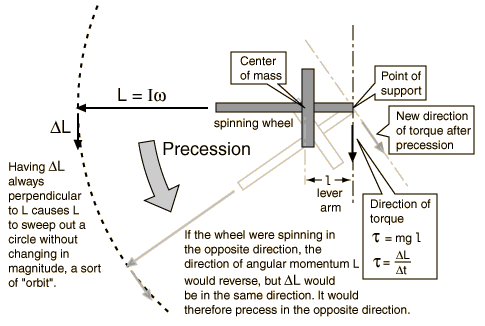Earth's rotational properties are best reflected by the motion of a gyroscope. A spinning gyroscope negates gravity and maintains a great rotational stability. Any free-spinning wheel (see drawing below) such as a bicycle wheel can become a gyroscope. It will rotate around an axis that can point in any direction while it spins. Once the wheel has a lot of angular momentum, it will float upright in air turning as it precesses. The torque is on the spin axis. The gyro precesses at a right angle to the direction of the torque. In the drawing, changes are indicated by a light yellow line. Observe the changes to the angular momentum (L is perpendicular to delta L). The wheel wobbles as the direction of the angular momentum changes by a change in velocity. Equation: Torque = Inertia x Angular Acceleration = Changed Angular Momentum divided by the Time Interval. The precession stops when the direction of the spin and the torque direction are in line. Image: Hyperphysics.phy-astr.gsu.edu Because a spinning body will align its axis perpendicular to a force, we can gather that Earth has a great rotational stability. Find more detailed information on gyroscopes and astrophysics at hyperphysics.phy-astr.gsu.edu For final thought move on to next page